how to find standard deviation of probability distribution

Probability Distribution Formula

Probability Distribution Formula (Table of Contents)

Formula
Examples
Calculator

What is the Probability Distribution Formula?

The term "probability distribution" refers to any statistical function that dictates all the possible outcomes of a random variable within a given range of values. One of the most common examples of a probability distribution is the Normal distribution. However, there are other major categories of probability distributions – Chi-square distribution, Binomial distribution, and Poisson distribution.

On the other hand, the term "probability distribution formula" covers the formula of parameters of a probability distribution – mean, standard deviation, skewness, and kurtosis. However, in this article, we will discuss the formula for mean and standard deviation.

The mean is the expected value of the random variable in the probability distribution. The formula for the mean of a probability distribution is expressed as the aggregate of the products of the value of the random variable and its probability. Mathematically, it is represented as,

x̄ = ∑ [x_i * P(x_i)]

where,

x_i = Value of the random variable in the i^th observation
P(x_i) = Probability of the i^th value

The standard deviation is a measure of the variation of all the random variable values from its expected value. The formula for standard deviation is expressed as the square root of the aggregate of the product of the square of the deviation of each value from the mean and the probability of each value. Mathematically, it is represented as,

ơ = √ ∑ (x_i– x̄)² * P(x_i)

Please note that the summation of all the probabilities in a probability distribution is equal to 1.

Examples of Probability Distribution Formula (With Excel Template)

Let's take an example to understand the calculation of the Probability Distribution Formula in a better manner.

You can download this Probability Distribution Formula Excel Template here – Probability Distribution Formula Excel Template

Probability Distribution Formula – Example #1

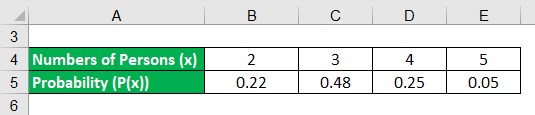
Let us take the example of a survey conducted in a certain to find out the expected number of persons in a family; the following data is available. Calculate the mean and standard deviation of the probability distribution.

Probability Distribution Formula-1.1

Solution:

Mean (x̄) is calculated using the formula given below

x̄ = ∑ [x_i * P(x_i)]

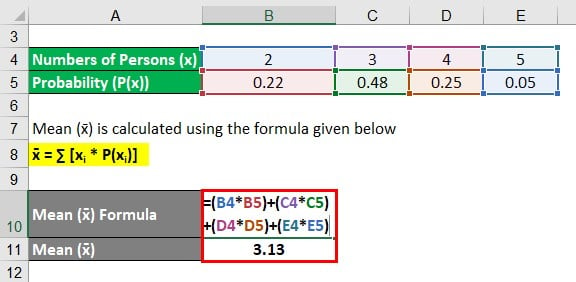
Probability Distribution Formula-1.2

Mean (x̄) = 2 * 0.22 + 3 * 0.48 + 4 * 0.25 + 5 * 0.05
Mean (x̄) = 3.13

Standard Deviation (ơ) is calculated using the formula given below

Standard Deviation (ơ)= √ ∑ (x_i– x̄)² * P(x_i)

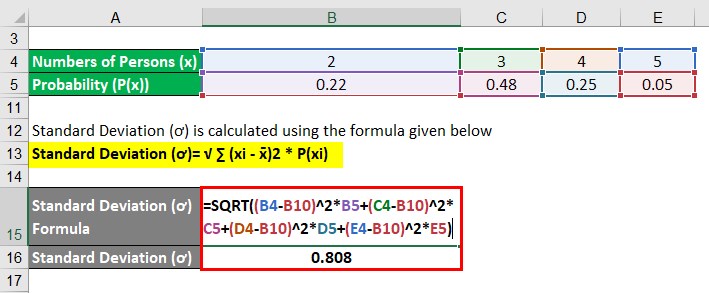
Probability Distribution Formula-1.3

Standard Deviation (ơ)=√ [(2 – 3.13)² * 0.22 + (3 – 3.13)² * 0.48 + (4 – 3.13)² * 0.25 + (5 – 3.13)² * 0.05]
Standard Deviation (ơ) = 0.808

Therefore, according to the survey, the expected no. of persons per family is 3.13 with a standard deviation of 0.808.

Probability Distribution Formula – Example #2

Let us take the example of a bag with 2 red balls and 4 blue balls. If two balls are drawn at random without replacement, then calculate the expected no. of red balls and their standard deviation.

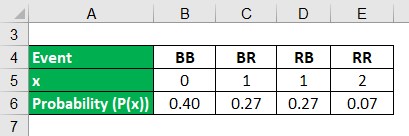
Probability Distribution Formula-2.1

Solution:

Mean (x̄) is calculated using the formula given below

Mean (x̄) = ∑[x_i * P(x_i)]

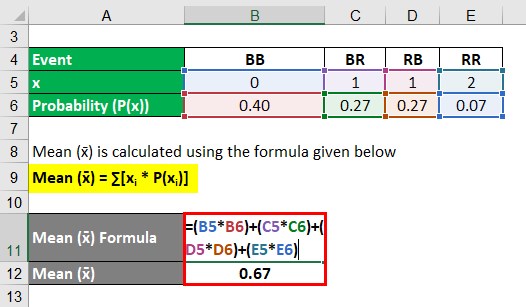
Mean (x̄) -2.2

Mean (x̄) = 0 * 0.40 + 1 * 0.27 + 1 * 0.27 + 2 * 0.07
Mean (x̄) = 0.67

Standard Deviation (ơ) is calculated using the formula given below

Standard Deviation (ơ) = √ ∑ (x_i– x̄)² * P(x_i)

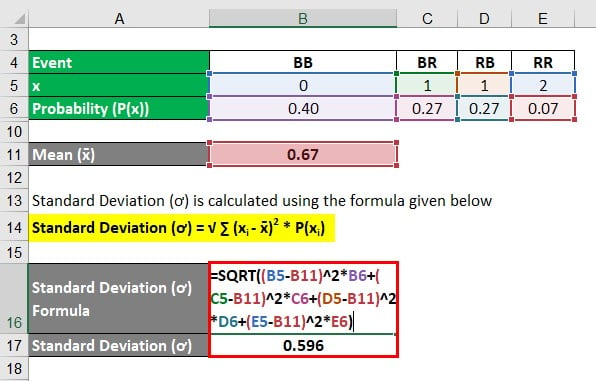
Standard Deviation (ơ) -2.3

Standard Deviation (ơ) = √ [(0 – 0.66)² * 0.40 + (1 – 0.66)² * 0.27 + (1 – 0.66)² * 0.27 + (2 – 0.66)² * 0.06]
Standard Deviation (ơ) = 0.596

Therefore, the expected no. of red balls, in this case, is 0.67 with a standard deviation of 0.596.

Explanation

The formula for a mean and standard deviation of a probability distribution can be derived by using the following steps:

Step 1: Firstly, determine the values of the random variable or event through a number of observations, and they are denoted by x₁, x₂, ….., x_n or x_i.

Step 2: Next, compute the probability of occurrence of each value of the random variable and they are denoted by P(x₁), P(x₂), ….., P(x_n) or P(x_i).

P(x_i) = No. of Events with i^th Value / Total No. of Events

Step 3: Next, the formula for mean can be derived by adding up the products of the value of the random variable (step 1) and its probability (step 2), as shown below.

x̄ = ∑[x_i * P(x_i)]

Step 4: Next, compute each value's deviation (step 1) of the random variable from the mean (step 3) of the probability distribution.

D_i = x_i – x̄

Step 5: Next, the formula for standard deviation can be derived by adding up the products of the squares of deviation of each value (step 4) and its probability (step 2) and then computing the square root of the result as shown below.

ơ = √[∑(x_i– x̄)² * P(x_i)]

Relevance and Use of Probability Distribution Formula

The probability distribution formula concept is very important as it basically estimates the expected outcome on the basis of all the possible outcomes for a given range of data. One of the most important parts of a probability distribution is the definition of the function, as every other parameter just revolves around it. Probability distribution finds application in the calculation of the return of an investment portfolio, hypothesis testing, the expected growth of population, etc.

Probability Distribution Formula Calculator

You can use the following Probability Distribution Formula Calculator

ơ	= √[∑(xi - x̄ )²* P(xi) ]
	√[∑(0 - 0 )²* 0 ] = 0